Question

`\underline{ \underline{ \text{question}}} :`

In the given figure , AP = BP = PC. Prove that
`\angle`ABC = 1 rt.angle.

~Thanks in advance ! ♡

Answer 1

this is your answer look it once.

Answer 2

See Below.

Explanation:

In the given figure, AP = BP = PC.

And we want to prove that ∠ABC is a right angle.

Since AP = BP and BP = PC, we can create two isosceles triangles: ΔAPB and ΔCPB.

By the definition of isosceles triangles, in ΔAPB, ∠PAB and ∠PBA are equivalent. Let the measure of each of them be x°.

Likewise, in ΔCPB, ∠PCB and ∠PBC are equivalent.

And since AP = BP = PC, each of the angles∠PCB and ∠PBC will also be equivalent to x°.

And since the sum of the interior angles of a triangle total 180°, we acquire:

`\angle PAB+\angle PBA+\angle PCB+\angle PBC=180`

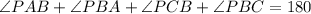
Since they are all equivalent:

`4x=180`

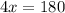
Hence:

`x=45^\circ`

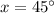
∠ABC is the sum of ∠PBA and ∠PBC, each of which measures 45°. Hence:

`\angle ABC=\angle PBA+\angle PBC=45+45=90^\circ`