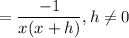Question

If g (x) = 1/x then [g (x+h) - g (x)] /h

Answer 1

`(-1)/(x(x+h)), h\\e 0`

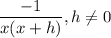
Explanation:

If
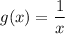`g(x) = (1)/(x)`, then
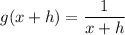`g(x+h) = (1)/(x+h)`. It follows that

`\begin{aligned} \\(g(x+h)-g(x))/(h) &= (1)/(h) \cdot [g(x+h) - g(x)] \\&= (1)/(h) \left( (1)/(x+h) - (1)/(x) \right)\end{aligned}`

Technically we are done, but some more simplification can be made. We can get a common denominator between 1/(x+h) and 1/x.

`\begin{aligned} \\(g(x+h)-g(x))/(h) &= (1)/(h) \left( (1)/(x+h) - (1)/(x) \right)\\&=(1)/(h) \left((x)/(x(x+h)) - (x+h)/(x(x+h)) \right) \\ &=(1)/(h) \left((x-(x+h))/(x(x+h))\right) \\ &=(1)/(h) \left((x-x-h)/(x(x+h))\right) \\ &=(1)/(h) \left((-h)/(x(x+h))\right) \end{aligned}`

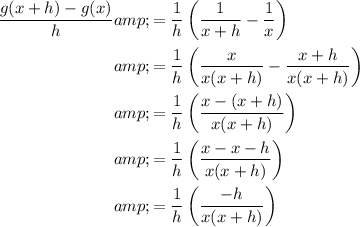
Now we can cancel the h in the numerator and denominator under the assumption that h is not 0.

`= (-1)/(x(x+h)), h\\e 0`