Answer: 1692
Explanation:
if prior estimate of proportion is not known , then the formula to find the sample size is given by:-
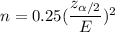 (1)
(1)
For 90% confidence interval , significance level =
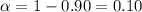
Critical value =
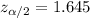
Margin error = 0.02
Now, substitute all the above values in (1), we get

Hence, the required minimum sample size should be 1692.