Question

If  


`x(t) = at + b(t)^(2) + c {t}^(3)`


Find the units of a, b and c. When( X) in m and (t) in sec.

Answer 1

If x(t) is measured in meters (m) and t is measured in seconds (s), then we must have
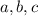`a,b,c` such that the units remain consistent:

`x\,\rm m=a(t\,\rm s)+b(t\,\rm s)^2+c(t\,\rm s)^3`

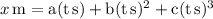
For this to happen, we need s to cancel in the first term and produce a length in m; for this, we would get a in m/s.

In the second term, s^2 needs to be canceled, so b has unit m/s^2.

In the third term, s^3, so c has unit m/s^3.