Answer:
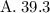
Explanation:
Let
 represent the height of the two smaller triangles in this figure. Because all three triangles are similar, we can set up the following proportion:
represent the height of the two smaller triangles in this figure. Because all three triangles are similar, we can set up the following proportion:
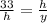 , for
, for
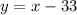 .
.
We can solve for
 using the Pythagorean theorem on the second largest triangle:
using the Pythagorean theorem on the second largest triangle:
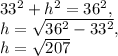 .
.
Now plugging in
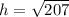 into our proportion
into our proportion
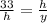 , we can solve for y:
, we can solve for y:
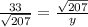 .
.
Cross-multiply to get:
 .
.
Since we
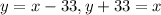 .
.
Therefore, our answer is:
 .
.