Question

Interpreting the Multiplicity of a Zero:

Determine the multiplicity of the roots of the function

Answer 1

GGGGGGGGGGGGGGGGGGGGGGGGGGGOOOOOOOOOOOOOOOOOOTTTTTTTTTTTTTTTTTTTAAAAAAAAAAAAAAAAAAA snatch a lil bit of points rq

Answer 2

For this case we have the following expression:

`k (x) = x (x + 2) ^ 3 (x + 4) ^ 2 (x-5) ^ 4\\`

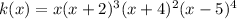
The roots are:

`x = -2\\\\x = -4\\\\x = 5\\\\x = 0\\`

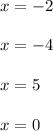
For example: For x = 0 we have

`k (0) = 0 (0 + 2) ^ 3 (0 + 4) ^ 2 (0-5) ^ 4\\\\k (0) = 0 (2) ^ 3 (4) ^ 2 (-5) ^ 4\\\\k (0) = 0 (8) (16) (625)\\\\k (0) = 0\\`

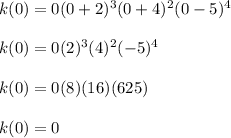
so it is shown that
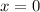`x = 0` is a root.

By definition, multiplicity represents the number of times a root is repeated in a polynomial, in turn it is given by the degree of the term that contains the root.

Thus:

The multiplicity of 0 is 1

The multiplicity of -2 is 3

The multiplicity of -4 is 2

The multiplicity of 5 is 4

Answer:

The multiplicity of 0 is 1

The multiplicity of -2 is 3

The multiplicity of -4 is 2

The multiplicity of 5 is 4