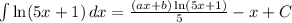Step-by-step explanation
The ii says "hence". This tells you that you must proceed from i above.
Given;
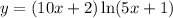
After differentiating in i, we had;
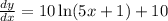
We now integrate both sides to obtain;

This gives,

We now split the integral to obtain;

This implies that,
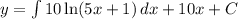
We rearrange to get,
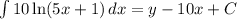
But
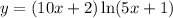
This implies,
10
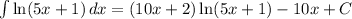
We divide through by 10.
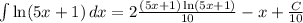
NB: The constant C divided by 10 is still a constant.
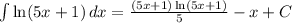
If a=5 and b=1 and we substitute, we get a general formula,but they were partially substituted to get.