Answer:
Since discriminant is positive, therefore, the given quadratic equation has two real solutions. Correct answer is the last option.
Explanation:
We have been given a quadratic equation and we need to figure out the number of real solutions of the equation.
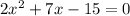
We know that discriminant of a general quadratic equation
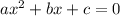 is given by
is given by
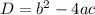
For the given quadratic equation
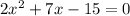 , we have
, we have
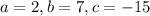 . Upon substituting these values in the formula for discriminant, we get:
. Upon substituting these values in the formula for discriminant, we get:
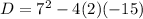
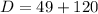

Since discriminant is a positive number, therefore, the given quadratic equation has two real solutions. Hence, the last option is the correct answer.