Question

A play is performed for a crowd of 400 people. Adult tickets cost $22 each, student tickets cost $15 each, and tickets for children cost $13.50 each. The revenue for the concert is $7840. There are 40 more children at the concert than students. How many of each type of ticket are sold?

Answer 1

280 adult tickets, 40 student tickets and 80 children tickets were sold.

Explanation:

To solve this problem let's call:

x = number of tickets for adults

y = number of tickets for students

z = number of tickets for children

The income for the concert is $ 7840

Then we can raise the following equations according to the given conditions:

`x+y+z = 400` (i)

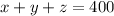`22x+15y +13.50z = 7840` (ii)

There are 40 more children in the concert than students:

So:

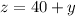`z=40+y` (iii)

We then have 3 equations and 3 unknowns:

To solve the system we multiply the equation (i) by -22 and add it to the equation (ii)

So:

`-22x-22y-22z=-8800`

+

`22x+15y +13.5z =7840`

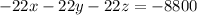
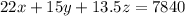
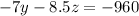`-7y-8.5z = -960` (iv)

Now we multiply (iii) by 7 and add it to (iv)

`7y-7z = -280`

+

`-7y-8.5z = -960`

`-15.5z = -1240`

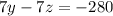
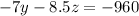
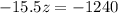
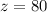`z = 80` (v)

We substitute (v) in (iv) and we have

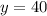`y = 40` (vi)

We substitute (v) and (vi) into (i) and we have:

`x =280`

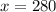
280 adult tickets, 40 student tickets and 80 children tickets were sold.