Answer:
Option: B is the correct answer.
The function is:
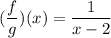
and the domain is: The set of all real numbers except 2
Explanation:
We are given a function f(x) and g(x) in terms of variable x by:
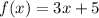
and
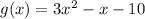
Now, the function
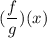 is given by:
is given by:

i.e.
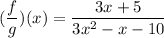
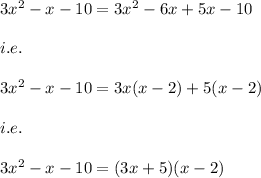
i.e.
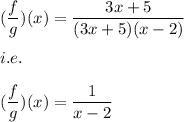
Also, the domain of the function is the set of all the real values except where the denominator is equal to zero.
The denominator is equal to zero when x=2.
Hence, the domain is the set of all the real values except 2.