Question

Order the steps to solve the equation log3(x + 2) = log3(2x^2 − 1) from 1 to 6.

0 = (2x − 3)(x + 1)
0 = 2x^2 − x −3
Potential solutions are −1 and 3/2
2x − 3 = 0 or x + 1 = 0
x + 2 = 2x^2 − 1
3^log3(x + 2) = 3^log3(2x2 − 1)

Answer 1

on Edg. the order of the answers would be 4,3,6,5,2,1

Answer 2

Consider the equation
`\log_3(x + 2)=\log_3(2x^2-1).`

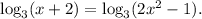
1 step (option 6): rise 3 to the power of left and right sides

`3^(\log_3(x + 2))=3^(\log_3(2x^2-1)),\\ \\x+2=2x^2-1.`

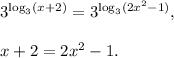
Thus, you get equation from option 5.

2 step (option 2): rewrite the equation in one side

`2x^2-x-2-1=0,\\ \\2x^2-x-3=0.`

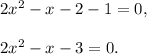
3 step (option 1): factor the previous equation

`D=(-1)^2-4\cdot 2\cdot (-3)=25,\ √(D)=5,\\ \\x_1=(1-5)/(4)=-1,\ x_2=(1+5)/(4)=(3)/(2),\\ \\2x^2-x-3=2\left(x-(3)/(2)\right)(x+1)=(2x-3)(x+1).`

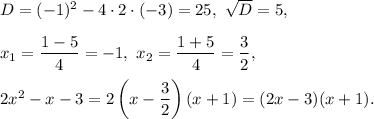
Then the equatios becomes

`(2x-3)(x+1)=0.`

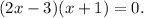
4 step (option 4): If product is equal to zero, then either
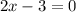`2x-3=0` or
`x+1=0.`

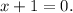
5 step (option 3): Then Potential solutions are −1 and 3/2. You can check them substituting into the initial equation:

for
`x=-1:`
`\log_3(-1+ 2)=\log_31=0,\\ \\ \log_3(2\cdot (-1)^2-1)=\log_3 1=0.`

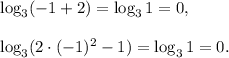
for
`x=(3)/(2):`
`\log_3\left((3)/(2)+ 2\right)=\log_3(7)/(2),\\ \\ \log_3(2\cdot \left((3)/(2)\right)^2-1)=\log_3 (7)/(2).`

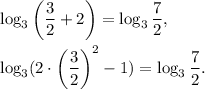
Both are roots.

Answer: correct order of steps is 6, 5, 2, 1, 4, 3.