Question

A biologist recorded a count of 337 bacteria present in a culture after 5 minutes and 699 bacteria present after 15 minutes.

To the nearest whole number, what was the initial population?

Answer 1

The initial population was 234.

Step-by-step explanation

Formula for the exponential growth is:
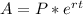`A= P*e^r^t` , where P is the initial amount, A is the final amount, r is the rate of growth and t is the time duration.

There was 337 bacteria after 5 minutes and 699 bacteria after 15 minutes. So, the equations will be......

`337=P*e^5^r .................................. (1)\\ \\ 699=P*e^1^5^r ................................. (2)`

Now dividing equation (2) by equation (1) , we will get .......

`(699)/(337)=(e^1^5^r)/(e^5^r) \\ \\ e^1^0^r = (699)/(337)`

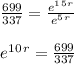
Taking 'natural log' on both sides.........

`ln (e^1^0^r) = ln ((699)/(337))\\ \\ 10r= 0.7295....\\ \\ r= 0.07295.... \approx 0.073`

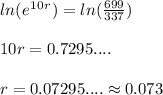
Now, plugging this
`r=0.073` into equation (1), we will get......

`337= P*e^5^(^0^.^0^7^3^)\\ \\ 337= P*1.4405 \\ \\ P= 233.946 \approx 234`

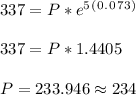
So, the initial population was 234.