Answer:

Explanation:
Given that,
 ...(1)
...(1)
We need to find the value of
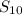 .
.
Put n = 1 to find the first term.

Put n = 2 to find the second term.
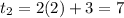
Put n = 3 to find the third term.
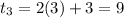
Put n = 10 to find the tenth term.
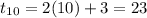
It means we need to find the sum of 5,7,9,.....,23.
The formula for the sum of n terms is given by :

We have, n = 10, a = 5 and
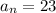
So,
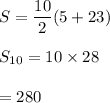
So, the value of
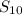 is equal to 280.
is equal to 280.