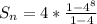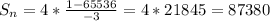Answer:
Sum is 87,380
Explanation:
the sum of the geometric sequence 4, 16, 64,...... 8 terms
To find the sum of geometric sequence use formula

a_1 is the first term
r is the common ratio
To find out common ratio 'r', divide the second term by first term
16/4= 4
64/16= 4
r= 4
first term is also 4
plug in the values in the formula