Question

Find an equation of the plane consisting of all points that are equidistant from (0, -2, 0) and (4, -3, 0), and having 4 as the coefficient of x.

Answer 1

The equation of the plane is 4x - 10y + 8 = 0.

Step-by-step explanation:

To find an equation of the plane, we need to determine the midpoint of the line segment connecting the two given points. The midpoint is (2, -2.5, 0).

Now, we can write the equation of the plane using the point-normal form: 4(x-2) - 2.5(y+2) + 0(z-0) = 0. Simplifying, we get 4x - 10y + 8 = 0.

Therefore, the equation of the plane consisting of all points that are equidistant from (0, -2, 0) and (4, -3, 0), and having 4 as the coefficient of x, is 4x - 10y + 8 = 0.

Answer 2

SOLUTION - GIVEN = Two Points (0,-2,0) & (4, -3,0 ) which are inequidistant

from the all the points of plane.

Find out = Equation of plane

TO PROOF - The general equation of plane .

a ( x-x₀) + b ( y- y₀) + c(z-z₀) =0

let A = (0,-2,0) & B = (4, -3, 0 )

is orthogonal to plane and become the normal vector.)

let O be midpoint
`\vec{AB}`

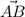
O =
`(1)/(2)`( 0+4, -2-3, 0-0 )

=\frac{1}{2} ( 4, -5, 0)

=
`(2, (-5)/(2),0)`

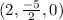
Equation of the plane by using the point & normal vector

by using general equation of a plane

4 ( x- 2) -1 (y +
`(5)/(2)` = 0

`image`

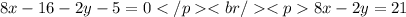
Hence Proved