Question

The manager of an accounting department wants to form a two

person advisory committee from the 18 employees in the department. In how many ways can the manager do this? Pls show work.

Answer 1

Answer: 153

Explanation:

When we have a group of N elements, the total number of combinations of K elements ( K ≤ N) is:

`C (N, K) = (N!)/((N-k)!*K!)`

Where N! = N*(N - 1)*(N - 2)*...*2*1

In this case, we have a group of 18 people (then N = 18) and we want to see how many different combinations of 2 we can make (K = 2).

Using the above equation we get:

`C(18, 2) = (18!)/((18 - 2)!*2!) = (18!)/(16!*2!) = (18*17)/(2) = 9*17 = 153`

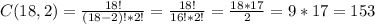
There are 153 different ways in which the manager can do this.