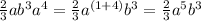Question

Can you teach me how to do these?? plzaa




thanksss!

Answer 1

if we are simplifying, ⅜ ab³a⁴ is
⅜a^5b³. when multiplying like bases you add exponents

second is xy²/2. when dividing like bases you subtract exponents

Answer 2

In general, you make use of the rules of exponents. It can be helpful to understand where they come from.

An exponent signifies repeated multiplication.

`x\cdot x\cdot x=x^(3)\qquad\text{the exponent 3 means x is a factor 3 times}`

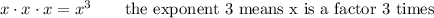
When you multiply, you add exponents.

`(x\cdot x\cdot x)* (x\cdot x)=(x\cdot x\cdot x\cdot x\cdot x)\\\\x^(3)* x^(2)=x^((3+2))=x^(5)`

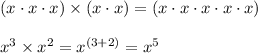
Likewise, when you divide, you subtract exponents. You can also think of this as adding the opposite of exponents that are in the denominator.

`(x\cdot x\cdot x)/(x\cdot x)=(x\cdot x)/(x\cdot x)* x=x\\\\(x^(3))/(x^(2))=x^((3-2))=x^(1)=x`

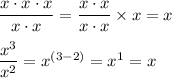
It should be no surprise then that if there are excess factors in the denominator, they can be expressed using a negative exponent.

`(x\cdot x)/(x\cdot x\cdot x)=(1)/(x)\\\\(x^(2))/(x^(3))=x^((2-3))=x^(-1)\qquad\text{using exponents}`

The idea of using multiplication to show repeated addition applies to exponents as well.

`(x\cdot x)* (x\cdot x)* (x\cdot x)=(x\cdot x\cdot x\cdot x\cdot x\cdot x)\\\\=x^(2)\cdot x^(2)\cdot x^(2)=x^((2+2+2))\\\\=\left(x^(2)\right)^(3)=x^(2\cdot 3)=x^(6)`

_____

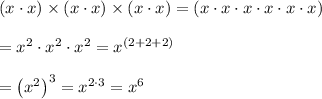
With these ideas in mind ...

`(2)/(3)ab^(3)a^(4)=(2)/(3)a^((1+4))b^(3)=(2)/(3)a^5b^3`

`(8xy^(8))/(16y^(6))=(8)/(16)xy^((8-6))=(1)/(2)xy^(2)`