Now despite what you may think, 1:3 is not 1/3 in this situation, but rather it's "1 equal part to 3 equal parts", which is a total of 4 equal parts. In short, they want the point that is at the 1/4 mark from A to B.
Firstly, how many units apart is 1 to 5 (the x-coordinates)? That would be 4 units apart. Multiply 1/4 by 4:
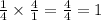
Next, how many units apart is 2 to 3 (the y-coordinates)? That would be 1 unit apart. Multiply 1/4 by 1:
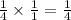
Next, since from 1 to 5 you are increasing, add 1 to 1.

2 is the x-coordinate of the new point.
Next, since from 2 to 3 you are increasing, add 1/4 to 2.
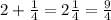
9/4 is the y-coordinate of the new point.
Putting it together, the point which divides AB into a 1:3 ratio is (2, 9/4), or D.