So firstly, the three terms share a GCF of x, so factor that out:

Next, I'm going to be factoring by grouping. But first, what two terms have a product of 140x^2 and a sum of 33x? That would be 28x and 5x. Replace 33x with 5x + 28x:
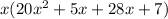
Now factor 20x^2 + 5x and 28x + 7 separately, make sure that they have the same quantity on the inside:
![x[5x(4x+1)+7(4x+1)]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/3ww8rhmizfrufcsp4442k03fyqbv6mvuql.png)
Now you can rewrite the expression as
![x[(5x+7)(4x+1)]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/tik3gbm6jy08fvc0uqzw5ya1n1x1rvmzab.png) , which is your final answer.
, which is your final answer.