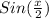Answer:
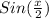
Explanation:
The period of a sine function is the angle measurement in radians in which the graph completes one complete cycle.
The period of a sin functio in its standard form is
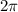
When the graph is stretched on x axis to a level that it now comletes one complete cycle in
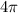 , there are changes in the angle whose sine has been taken
, there are changes in the angle whose sine has been taken
Which is given by the rule which says
The period of

Hence the function having
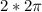 as its period, will be
as its period, will be