Answer:
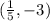 .
.
Explanation:
We have been given the coordinates of points A(-3,-5) and B (5,0). We are asked to find the coordinates of the point P on a directed line segment AB that partitions AB in the ratio 2:3.
We will use section formula to solve our given problem. When a point P divides a line segment internally in ratio m:n, then coordinates of point P are:
![[x=(m\cdot x_2+n\cdot x_1)/(m+n);y=(m\cdot y_2+n\cdot y_1)/(m+n)]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/sacw9rj1vypub4hy85hyzy1up8e8zg5k1n.png)
Upon substituting coordinates of our given points in section formula we will get,
![[x=(2\cdot 5+3\cdot -3)/(2+3);y=(2\cdot 0+3\cdot -5)/(2+3)]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/3cuzijfqunahnve1k80nt5lskdl5d6kcko.png)
![[x=(10-9)/(5);y=(0-15)/(5)]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/561cwtszidy834xscbg9ohd98j71e3h13v.png)
![[x=(1)/(5);y=(-15)/(5)]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/puf1rr305efn8wcjix7ctlwwvpo5zzgamo.png)
![[x=(1)/(5);y=-3]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/uay2ikmlxnl0bgucyqv94ejlkmpy21ywot.png)
Therefore, the coordinates of point P are
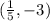 .
.