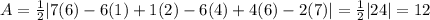Question

What is the area of triangle pqr on the grid? a triangle pqr is shown on a grid. the vertex p is on ordered pair 7 and 6, vertex q is on ordered pair 1 and 6, and the vertex r is on ordered pair 4 and 2. 5 square units 6 square units 10 square units 12 square units?

Answer 1

12

Explanation:

Answer 2

P(7,6), Q(1,6), R(4,2)

We have PQ parallel to the x axis. We'll call that the base,

b = 7 - 1 = 6

The altitude is then the y difference h = 6 - 2 = 4

The area is
`\frac 1 2 bh = \frac 1 2 (6)(4) = 12`

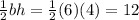
Answer: 12 square units

In general we can use the shoelace formula for the area of any polygon given coordinates. We write the points like this:

(7,6), (1,6), (4,2)

(1,6), (4,2), (7,6)

The area is then half the absolute value of the sum of the cross products:

`A = \frac 1 2 | 7(6)-6(1) + 1(2)-6(4) + 4(6)-2(7) | = \frac 1 2 |24| = 12`