For similar triangles
The ratio of the areas of the triangle is equal to the square of the ratio of the corresponding sides.
We know two equilateral are always similar.
Now it is given that area of ΔABC = 4 × ΔPQR
So
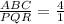
But according to the rule of similarity
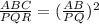
So we have

Taking square root on both sides
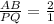
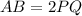
So each side of ΔABC is two times the side of ΔPQR.