Question

Type the correct answer in the box. Use numerals instead of words. If necessary, use / for the fraction bar.

In ΔPRQ, the length of is 8 units. The length of mid-segment XY is
units.

Answer 1

4

Explanation:

Answer 2

From given picture we can see that X is mid point of line PQ

So PQ=2PX

From given picture we can see that Y is mid point of line PR

So PR=2PY

Now consider triangles PRQ and PYX

Sides PQ and PX has same ratio as of sides PR and PY (as calculated above)

angle XPY= angle QPR (common angle)

Hence triangles PRQ and PYX are similar.

We know that ratio of the sides of similar triangles is always equal so we can write:

`(PQ)/(PX) = (QR)/(XY)`

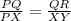
Plug the given values QR=8 and PQ=2PX

`(2PX)/(PX) = (8)/(XY)`

`(2)/(1) = (8)/(XY)`

`2 = (8)/(XY)`

`XY = (8)/(2)`

`XY = 4`

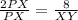
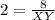
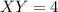
Hence final answer is XY = 4 units.