Question

Find the discriminant of the equation. x2 + 3x - 4 = 0

Answer 1

Please use " ^ " to indicate exponentiation: x^2 + 3x - 4 = 0

Here, a = 1, b = 3 and c = -4.

The formula for the discriminant is b^2 - 4(a)(c).

Substituting the given values of a, b and c, we get:

(3)^2 - 4(1)(-4)

Evaluating this, we get 9 + 16 = 25.

The discriminant is 25.

-3 plus or minus √25

Taking this further, x = ------------------------------------

2

-3 plus or minus 5

or: x = -------------------------------- => {-4, 1} (solutions)

2

Answer 2

With a standard form quadratic, we see that with the quadratic formula:

`(-b\pm√(b^2-4ac))/(2a)`

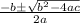
That the part under the radical (
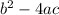`b^2-4ac`) can tell us many things about how the roots of this polynomial behave.

If b^2-4ac were 0, that would mean the polynomial would have one root at
`(-b)/(2a)`. We call that a double root at (-b/2a,0).

If the discriminant is a perfect square, we note that the radical can be reduced and this the fraction is a rational number.

If the discriminant is negative, it means it has no real roots. That's because the root of a negative number is imaginary.

The last case if is the discriminant is neither a negative or a perfect square. That means the radical cannot be reduced and we will have two irrational roots.

In this problem, we see that the discriminant is:

`3^2-4*1(-4)= \\9+16=\\25`

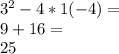
The answer to the problem you asked is then 25, and we can also note that this quadratic has real, rational roots.