Question

Which statement is correct with respect to f(x) = -3|x − 1| + 12?

A.
The V-shaped graph opens upward, and its vertex lies at (-3, 1).

B.
The V-shaped graph opens downward, and its vertex lies at (-1, 3).

C.
The V-shaped graph opens upward, and its vertex lies at (1, -12).

D.
The V-shaped graph opens downward, and its vertex lies at (1, 12).

Answer 1

Let's start from the parent function
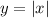`y = |x|` and see which transformation we have applied. The parent function's graph is open upards, and its vertes lies at
`(0,0)`

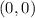
The first transformation is
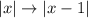`|x| \to |x-1|`. This is a tranformation of the form
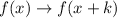`f(x)\to f(x+k)`. This kind of transformation shift the graph horizontally, k units to the left if k is positive, k units to the right if k is negative. In this case
`k = -1`, so the function is shifted one unit to the right. The new vertex lies at
`(1,0)`

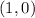
The second transformation is
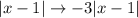`|x-1| \to -3|x-1|`. This is a tranformation of the form
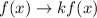`f(x)\to kf(x)`. This kind of transformation stretch the graph vertically, compressing it if k is between 0 and 1, expanding it if k is greater than 1. Moreover, if k is negative, the function is reflected along the x axis. In this case
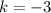`k = -3`, so the function is reflected and stretched. This means that now the graph opens downwards, the vertex still lies at
`(1,0)`.

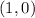Finally, we have
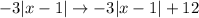`-3|x-1| \to -3|x-1|+12`. This is a tranformation of the form
`f(x)\to f(x)+k`. This kind of transformation translates the graph vertically, k units up if k is positive, k units down if k is negative. In this case,
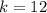`k = 12`, so the graph is shifted 12 units up. The graph still opens downwards, while the new vertex lies at
`(1,12)`

Answer 2

The answer should be D if not it is C