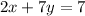Question

write an equation of the line containing the specified point and perpendicular to the indicated line (-4,5) 7x-2y=1

Answer 1

Equation of a line given
`7x-2y = 1`

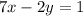
We have to find an equation of a line which is perpendicular to the given line.

If the general equation of a line is
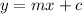`y=mx+c`, m is the slope of the line there. And the slope of the perpendicular line will be the negative reciprocal of m which is
`(-(1)/(m))`.

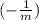So first here we have to make the given equation as
`y=mx+c`

`7x-2y = 1`

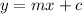
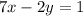
First we have to move 7x to the right side by subtracting it from both sides.

`-7x+7x-2y =-7x+1`

`-2y = -7x+1`

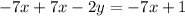
Now to get y we have to move -2, by dividing it on both sides.

`((-2y))/((-2)) = ((-7x+1))/((-2))`

`y = ((-7x))/((-2)) + (1)/((-2))`

`y= ((7)/(2) )x - (1)/(2)`

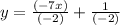
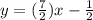
So here the slope
`m= (7)/(2)`

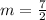
Now the slope for the perpendicular equation is

`-(1)/(m)= -(1)/((7/2))`

`-(1)/(m) = -(2)/(7)`

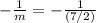
So slope of the perpendicular line is
`-(2)/(7)`

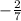
We can write the perpendicular equation as

`y=(-(2)/(7) )x+c`

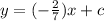
Now this equation is passing through the point (-4,5)

We have to plug in x = -4 and y = 5 in the line to get c.

`5= (-(2)/(7))(-4) + c`

`5= (8)/(7) +c`

`5-(8)/(7) = c`

`(35)/(7) -(8)/(7) =c`

`((35-8))/(7) = c`

`(27)/(7) =c`

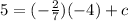
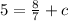
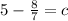
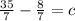
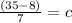
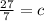
So we have got the value of c. Now we can write the perpendicular equation as,

`y= (-(2)/(7))x+(27)/(7)`

`y = ((-2x+27))/(7)`

`7y = -2x+7`

`7y+2x = -2x+2x+7`

`2x+7y = 7`

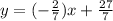
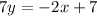
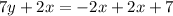
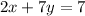
So we have got the required perpendicular line.

The equation of the perpendicular line is
`2x+7y = 7`