Question

The endpoints of `bar(EF)` are E(xE , yE) and F(xF , yF). What are the coordinates of the midpoint of `bar(EF)`?

A.
`((x_F)/(2),(y_F)/(2))`

B.
`((x_E)/(2),(y_E)/(2))`

C.
`((x_E+x_F)/(2),(y_E+y_F)/(2))`

D.
`(x_E+(x_F)/(2),y_E+(y_F)/(2))`

Answer 1

For a better understanding of the solution provided here please find the diagram attached.

Please note that in coordinate geometry, the coordinates of the midpoint of a line segment is always the average of the coordinates of the endpoints of that line segment.

Thus, if, for example, the end coordinates of a line segment are
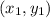`(x_(1), y_1)` and
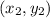`(x_2, y_2)` then the coordinates of the midpoint of this line segment will be the average of the coordinates of the two endpoints and thus, it will be:

`(((x_1+x_2))/(2), ((y_1+y_2))/(2))`

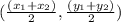
Thus for our question the endpoints are
`(x_E, y_E)` and
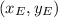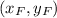`(x_F, y_F)` and hence the midpoint will be:

`(x_M, y_M)=`
`(((x_E+x_F))/(2), ((y_E+y_F))/(2))`

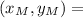
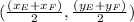
Thus, Option C is the correct option.