Question

Algebra 1 question 4.

Answer 1

Greetings!

To start this problem, let's first assign a variable for the missing, consecutive odd numbers. Since they are consecutive and odd, we add two.

Proof: 3-1=2, 5-3=2

The first, consecutive, odd number:
`x`

The second, consecutive, odd number:
`x+2`

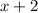
The third, consecutive, odd number:
`x+4`

The fourth, consecutive, odd number:
`x+6`

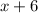
The sum of the values are equal to 3 times the sum of the first two numbers, of which this is equal to 35 less than the fourth number. Let's create an equation to simplify this:

`3((x)+(x+2))=(x+6)-35`

Complete the operations inside the parenthesis:

`3(2x+2)=(x+6)-35`

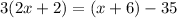
Distribute the parenthesis (utilizing the distributive property)

`(((2x)(3))+((2)(3)))=(x+6)-35`

`(6x+6)=(x+6)-35`

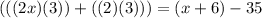
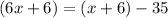
Simplify both sides:

`6x+6=x-29`

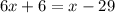
Add -6 and -x to both sides of the equation:

`(6x+6)+(-6)+(-x)=(x-29)+(-6)+(-x)`

`5x=-35`

Divide both sides of the equation by 5:

`(5x)/(5)=(-35)/(5)`

`x=-7`

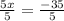
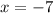
If
`x` is equal to -7:

`x+2=-5`

`x+4=-3`

`x+6=-1`

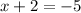
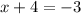
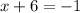
The four numbers are:

`\boxed{-7,-5,-3,-1}`

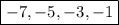
I hope this helps!

-Benjamin

Answer 2

1st integer - 2n+1

2nd integer - 2n+3

3rd integer - 2n+5

4th integer - 2n+7

`3(2n+1+2n+3)=2n+7-35\\3(4n+4)=2n-28\\12n+12=2n-28\\10n=-40\\n=-4\\\\2n+1=-7\\2n+3=-5\\2n+5=-3\\2n+7=-1`

The integers are: -7,-5,-3,-1