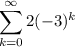Question

Write the sum using summation notation, assuming the suggested pattern continues.

2-6+18-54+...

Answer 1

Each term seems like it's -3 times the previous one, first term 2.

`\displaystyle \sum_(k=0)^(\infty) 2 (-3)^k`

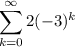
Going to infinity like that it's of course divergent, but perfectly summable if we replace infinity with a finite bound like n-1:

`\displaystyle \sum_(k=0)^(n-1) 2 (-3)^k`

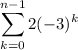
But, assuming the pattern continues means I'd go with infinity.

Answer:
`\displaystyle \sum_(k=0)^(\infty) 2 (-3)^k`