Question

The function g(n) = n^2 − 6n + 16 represents a parabola.

Part A: Rewrite the function in vertex form by completing the square. Show your work. (6 points)
Part B: Determine the vertex and indicate whether it is a maximum or a minimum on the graph. How do you know? (2 points)
Part C: Determine the axis of symmetry for g(n). (2 points)

Answer 1

well, lemme post a quick on how a perfect square trinomial works

as you should already know, now let's look at this equation, and let's start by grouping the variables,

g(n) = n² - 6n + 16

g(n) = (n² - 6n) + 16

g(n) = (n² - 6n + [?]²) + 16

so, we have a missing fellow there, in order to get a perfect square trinomial, well, the tell-tale guy is the middle term, the middle term is the product of 2 and the other two guys without the ², and we know in this case is 6n.

`\bf \stackrel{\stackrel{middle}{term}}{6n}=2(n)[?]\implies \cfrac{6n}{2n}=[?]\implies 3=[?]`

aha!!, there's our missing fellow, is 3.

well, let's keep in mind that all we're doing is borrowing from our very good friend Mr. Zero, 0, so if we add 3², we also have to subtract 3².

`\bf g(n)=(n^2-6n+3^2-3^2)+16\implies g(n)=(n^2-6n+3^2)+16-3^2\\\\\\g(n)=(n-3)^2+16-9\implies \boxed{g(n)=(n-3)^2+7}\\\\\\~~~~~~\textit{parabola vertex form}\\\\y=a(x- h)^2+ k\qquad\qquadvertex~~(\stackrel{}{ h},\stackrel{}{ k})\\\\\\g(n)=\stackrel{a}{1}(n-\stackrel{h}{3})+\stackrel{k}{7}\qquad vertex~(3,7)`

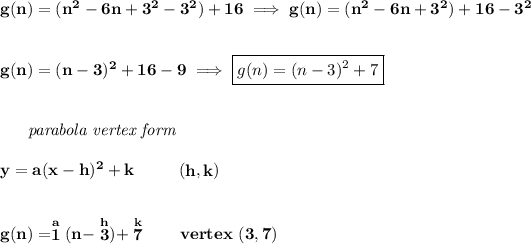
is it a maximum of a minimum? well, all we have to do is look at the leading term's sign, n² has a positive coefficient, that means the parabola is opening upwards, assuming "n" is drawn on the x-axis, and since it's opening upwards like a cup, it comes down down down, reaches a minimum and goes back up up up.

well, the axis of symmetry will just be the x-coordinate of the vertex, that is the line that will cut the parabola in two twin halves, namely x = 3.