Question

The path of a ball is given by y=-1/20x^2+3x+5, where y is the height in feet and x is the horizontal distance in feet. Find the maximum height of the ball and the horizontal distance at that height

Answer 1

The path of the ball is given by
`y=(-1)/(20)x^(2)+3x+5` where y is the height in feet and x is the horizontal distance in feet.

We will use the second derivative test which states,

If a function has a critical point for which f′(x) = 0 and the second derivative is positive at this point, then f has a local minimum. If, the function has a critical point for which f′(x) = 0 and the second derivative is negative at this point, then f has local maximum.

Firstly, we will calculate the first derivative of the given function,

`y'=(-2x)/(20)+3`

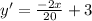
To find the horizontal distance, let y'=0

`(-2x)/(20)+3=0`

`(-2x)/(20)=-3`

`{-2x} =-60`

`x=30`

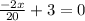
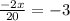
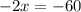
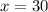
Now let us consider the second derivative of the given function,

`y''=(-2)/(20)`

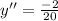
Since the second derivative that is y'' is negative.

Therefore, x=30 is the maximum horizontal distance.

Substituting x =30 in the given function to get the maximum height,

`y=(-1)/(20)x^(2)+3x+5`

`y=(-1)/(20)(30)^(2)+3(30)+5`

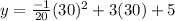
y=50 is the maximum height in feet.