Answer:
the degree of the polynomial
Explanation:
Given
Polynomial equation
Required
What does the sum of the multiplicities add up to
To answer this question, I'll make use of the following polynomial.
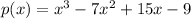
When factorized, the polynomial is:
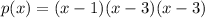

x-1 can be expressed as

So, we have:
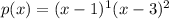
The sum of multiplicity (M) of the equation is 3.
This is so because
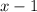 occurred one time in
occurred one time in

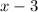 occurred two times in
occurred two times in

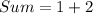
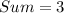
The degree of
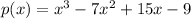 or
or
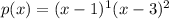 is 3.
is 3.
This implies that:
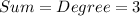
Hence: Option (a) answers the question