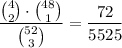Question

part c find the probability of drawing a three-card hand that includes two aces from a deck of 52 cards

Answer 1

We're drawing 3 cards from a deck of 52, and we have

`\dbinom{52}3=(52!)/(3!(52-3)!)`

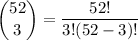
ways of drawing any 3-card hand.

Of the 4 total aces in the deck, we want to draw 2. The third card can be any 1 of the 48 remaining cards. We have

`\dbinom42\cdot\dbinom{48}1=(4!)/(2!(4-2)!)\cdot(48!)/(1!(48-1)!)`

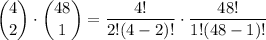
possible 3-card hands that contain any 2 aces.

The probability of drawing such a hand is then

`\frac{\binom42\cdot\binom{48}1}{\binom{52}3}=(72)/(5525)`