Answer:
Mike deposits $11 more each month.
Explanation:
Since, every month Mike is paying more money then the amount of money deposited can be in the form of an Arithmetic Progression.
First term, a = 9
Total number of months, n = 41
Total sum of money, Sn = $9389
We need to find amount which Mike is paying more every month that is d or common difference of the resulting A.P.
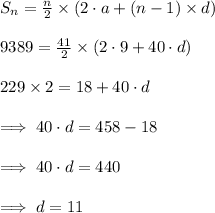
Hence, Mike deposits $11 more each month.