First question:
First of all, you have to rewrite the quadratic equation bringing everything to the left hand side, so you have
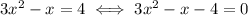
Now, the coefficients of a quadratic equation are usually read as
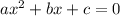 , i.e.
, i.e.
 is the coefficient of
is the coefficient of
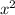 ,
,
 is the coefficient of
is the coefficient of
 and
and
 is the constant term.
is the constant term.
Once you rewrite your equation, the coefficient of
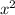 is 3, the coefficient of
is 3, the coefficient of
 is
is
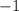 and the constant term is
and the constant term is
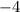 , so the correct answer is D.
, so the correct answer is D.
Second question:
As discussed above, the first step for solving a quadratic equation is bringing everything to the left, so that you are in the form
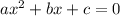 . So, the first thing you have to do is to transform
. So, the first thing you have to do is to transform
