Question

Please answer ASAP. I cannot get this right no matter how carefully I try to solve it lol

Answer 1

When we get a question with "probability of at least two the same" we should think of it as 1 minus the probability of all different.

Rather than dazzle you with chooses and factorials let's work our way up to it.

If there was only one lady, there's probability 1 no two will choose the same style.

With two ladies, the second has a 13/14 th chance of choosing a different coat.

With three ladies , the probability of all different is
`(13)/(14) \cdot (12)/(14)`

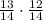
With eight ladies, we get seven fractions. We'll do 1 minus to get "two or more the same":

`p = 1 - (13)/(14) \cdot (12)/(14)\cdot \frac {11}{14} \cdot (10)/(14) \cdot (9)/(14) \cdot (8)/(14) \cdot (7)/(14) = 1 - (13!/6!)/14^7 \approx 0.918`

Second choice

5 boys 12 watch styles

P(Two boys different),=11/12

P(Three boys different),=11/12 * 10/12

P(Five boys different) = 11*10*9*8/12^4 = .3819

P(two of five match) = 1 - 11*10*9*8/12^4 = .6181

last choice