Hi there!
The 1ˢᵗ Step is to develop a relation between cost and time (in years).
∵ th' cost increases linearly, we know that the relation can be represented by the equation of a straight line, y = mx+b
where,
• y is the cost
• m is the rate of cost increase
• x is the year
• b is the initial cost of the time frame being analyzed.
∵ We're looking at the time between 1985 and 1995 :-
The initial cost will be the cost of the clothing in 1985, which is $620.
Next, the rate of increase of the cost can be found by the rise-over-run method.
∵ y is the cost and x is the time in years, the rise will be the change in cost and the run will be the change in time (years).
:-
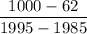
= $ 37.5 per year increase
Finally, th' relation:
y = 37.5x + 620
y = 37.5 × 6 + 620
y = $ 845
Hence,
The cost of family's clothing in 1991 is $ 845.
~ Hope it helps!