Question

A random sample of 121 automobiles traveling on an interstate showed an average speed of 73 mph. from past information, it is known that the standard deviation of the population is typically 11 mph. the 95% confidence interval average speed on this section of the interstate is:

Answer 1

121 is big enough to assume normality and not worry about the t distribution. By the 68-95-99.7 rule a 95% confidence interval includes plus or minus two standard deviations. So 95% of the cars will be in the mph range

`(73 - 2 \cdot 11, 73 + 2 \cdot 11) = (51,95)`

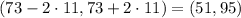
The question is a bit vague, but it seems we're being asked for the 95% confidence interval on the average of 121 cars. The 121 is a hint of course.

The standard deviation of the average is in general the standard deviation of the individual samples divided by the square root of n:

`\sigma = ( 11)/(√(121)) = 1`

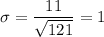
So repeating our experiment of taking the average 121 cars over and over, we expect 95% of the averages to be in the mph range

`(73 - 2 \cdot 1, 73 + 2 \cdot 1) = (71,75)`

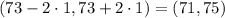
That's probably the answer they're looking for.