Question

F(x)=4x^2+1 and g(x)=x^2-5 fins (f+g)(x)

Answer 1

Hi there!


`(f +g )(x) = f(x) +g (x)`
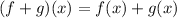
In other words: to find (f + g)(x) we must add up the functions f(x) and g(x). Substitute both functions into the formula.


`(f + g)(x) =( 4x {}^(2) + 1) + ( {x}^(2) - 5)`
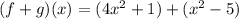
Remove the parenthesis, since they don't have a function in this situation.


`(f + g)(x) =4x {}^(2) + 1 + {x}^(2) - 5`
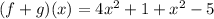
Rearrange the expression in order to collect terms later.


`(f + g)(x) = 4x {}^(2) + {x}^(2) + 1 - 5`
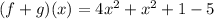
And finally collect the terms.


`(f + g)(x) = 5x {}^(2) - 4`
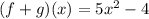
~ Hope this helps you!

Answer 2

`(f+g)(x)=4x^2+1+x^2-5=5x^2-4`