Question

the length of the rectangle is 3 ft less than double the width, the area of the rectangle is 44 ft squared. what is the length and the width?

Answer 1

A=wl is the area of the rectangle but here’s a picture to show u this might help u with the length and width have a wonderful and nice day

Answer 2

We need to find the length, l, and the width, w. Let's set up the two equations with the information found in the question:

l = 2w - 3
w = w
A = 44
`ft^(2)`

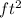
We know that area is equal to length * width (
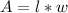
`A=l*w`), so let's substitute those values


`A=l*w`

`A=(2w-3)*w`

`A=2 w^(2)-3w`

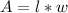
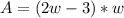
And we know that the area is equal to
`44 ft^(2)`:


`44=2 w^(2)-3w`

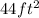
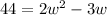
Now we set equal to 0 and factor in order to get w:


`0=2 w^(2)-3w-44`

`0=(2w-11)(w+4)`

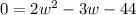
Then we set each factor equal to zero and solve for w. We get the two values:


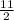
`(11)/(2)` and
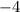
`-4` for w.

We know that the width cannot be negative, so we take the value that is positive,
`(11)/(2)`

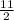
So now we know that w =
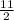
`(11)/(2)`, so we plug in this value into the equation for the length:


`l=2w-3`

`l=2( (11)/(2))-3`

`l= (22)/(2)-3`

`l=11-3=8`

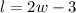
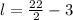
So we now know that the length is 8, and the width is
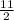
`(11)/(2)`.