The formula for the average value of a function is
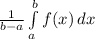
where b is the upper bound and a is the lower. For us, this formula will be filled in accordingly.

. We will integrate that now:
![(1)/(2)[ (2x^3)/(3)+3x]](https://img.qammunity.org/2019/formulas/mathematics/college/5zldldagt975euq96bcqnu61uxf5obh154.png)
from 0 to 2. Filling in our upper and lower bounds we have
![(1)/(2)[( (2(2^3))/(3)+3(2))-0]](https://img.qammunity.org/2019/formulas/mathematics/college/n1spy2am2htfxoqfjd6htqkfaeee37qis7.png)
which simplifies to
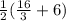
and
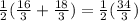
which is 17/3 or 5.667