Sure! So if you want to find the area under a curve, this calls for an
integral. I am assuming you know what that is, since this looks like a calculus question.
Since we cant to find the area under y=(x+1)^3 from -1 to 1, we can write the integral as "the integral of (x+1)^3 from -1 to 1", or seen as
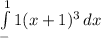
You can then plug this into your calculator and find it to be 4
The (maybe) geometry way:
We can separate this graph into two parts: a triangle and a trapezoid using the dotted lines. Then, we can find the area of the triangle and trapezoid and add them together. The area of the triangle is 1/2(b)(h). The base is 1 and the height is -1, so therefore, the area of the triangle is -1/2.
The area of a trapezoid is 1/2(a+b)*h. The height is 1, the
a (top part) is 1, and the
b (bottom part) is 8. Therefore, we plug that in and get 4.5
4.5+(-0.5)=4