Question

Choose the numbers that are irrational 1/3, 2/7, 2 in the house thingy, 3.624, 0.6 repeat, 0.87 repeat, -5

Answer 1

All the numbers listed are rational, since they can all be represented as a fraction, EXCEPT "2 in the house thingy", which I suppose is
`√(2)`.
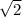
This last one is irrational, because it cannot be represented as a finite fraction.

The following is a check to make sure:
1/3 is already a fraction
2/7 is already a fraction
2 in the house thingy =
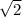
`√(2)` cannot be converted into a finite fraction.
3.624 = 3624/1000 is a fraction
0.6 repeat = 2/3 is a fraction
0.87 repeat = 87/99 = 29/33 is a fraction
-5 = -5/1 is a fraction.

Answer 2

⇒A number is said to be rational , if it can be written in the form of
`(p)/(q)` , where, q≠0,otherwise it is Irrational.

⇒The Decimal representation of Rational number is either terminating or Non terminating repeating.

⇒The decimal representation of Irrational number is Non terminating non repeating.

`1.\rightarrow (1)/(3)=\text{Rational}\\\\2.\rightarrow (2)/(7)=\text{Rational}\\\\3.\rightarrow 2 \text{in house thingy}=√(2)=\text{Irrational}\\\\4.\rightarrow 3.624=\text{Rational}\\\\5.\rightarrow 0.\overline{6}=\text{Rational}\\\\6.\rightarrow 0.\overline{87}=\text{Rational}\\\\7.\rightarrow -5=\text{Rational}`

⇒All numbers are rational, except "2 in the house thingy" which is Irrational.