Answer:
The model is a good fit for the data in the table because the residual plot has a Random pattern of data points about the horizontal axis.
Explanation:
Residual is the distinction between the dependent variable's observed value and the predicted value.
A linear regression model is good If the points of residual plot are spread randomly around the horizontal axis.
Consider the provided data,
Plot the x and y to make a residual plot and then draw the graph of the model:
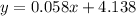
The required graph is shown in figure 1:
Using the above definition we can say that:
The model is a good fit for the data in the table because the residual plot has a Random pattern of data points about the horizontal axis.