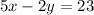Answer:
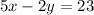
Explanation:
The equation of a line passing through (a,b) and (c,d) is given by :-
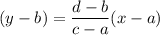
Also , the equation of a line in standard form is given by :-
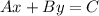
Then , the equation of a line passing through points (3,-4) and (5,1) will be :-

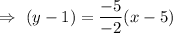
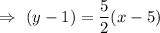
Convert into standard form.

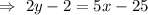
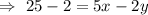
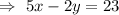
Hence, the standard form of the line passing through the points (3,-4) and (5,1) :