To solve this, we need to multiply each term in the first parenthesis with every term in the second parenthesis.
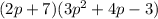
First, we multiply each part, starting with 2p and then going to 7, pairing them with each term in the second part.
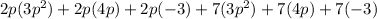
Then, we multiply the terms together.
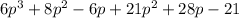
Lastly, we simplify the answer down by combining like terms.
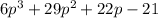
The third option in this case is the correct one.