Question

Which graph represents the function f(x)=4/x

Answer 1

The graph of given function is shown below.

Explanation:

The given function is

`f(x)=(4)/(x)`

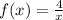
We need top find the graph of given rational function.

First find the key features of the given function.

1. Vertical asymptote: Equate denominator equal to 0.

`x=0`

The vertical asymptote is x=0.

2. Horizontal asymptote: Find the
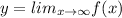`y=lim_(x\rightarrow \infty)f(x)` to find the horizontal asymptote.

`y=lim_(x\rightarrow \infty)f(x)=lim_(x\rightarrow \infty)(4)/(x)`

`y=(4)/( \infty)`

`y=0`

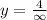
The horizontal asymptote is y=0.

3. The graph has vertical asymptote is x=0 and horizontal asymptote is y=0, therefore the graph has no x- and y-intercepts.

4. End behavior:

`f(x)\rightarrow 0\text{ as }x\rightarrow -\infty`

`f(x)\rightarrow -\infty\text{ as }x\rightarrow 0^-`

`f(x)\rightarrow \infty\text{ as }x\rightarrow 0^+`

`f(x)\rightarrow =\text{ as }x\rightarrow \infty`

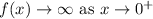
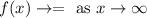
5. Table of value:

The table of values is

x f(x)

-2 -2

-1 -4

1 4

2 2

Plot these ordered pairs on a coordinate and connect these points by free hand curve using the key features.

Answer 2

Answer with explanation:

The given function is:

`f(x)=(4)/(x)`

→Domain and Range of the function is ,all real number excluding 0, which can be written as, x ∈ R→{0} and y∈ R→{0}.

So, if we replace , f(x) by, y ,the equation of function will become

x y = 4,which is rectangular Hyperbola.

→Horizontal Asymptote

`y=\lim_(x \to \infty) (4)/(x)=0`

→Vertical Asymptote

`x=\lim_(y \to \infty)(4)/(y)=0`