We'll use rise over run to find the slopes of two of the sides of the triangle:
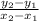
Because C is the rightmost point, we'll have it represent (x1,y1), and have A and B represent (x2,y2):
A to C
(-2,3) to (2,-1)
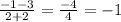
The slope of the line from A to C is -1.
B to C
(-3,-6) to (2,-1)
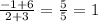
The slope of the line from B to C is 1.
These lines intersect at (2,-1), and they have negative reciprocal slopes of each other, which creates a perpendicular angle. This means that triangle ABC is a right triangle, and angle ACB is the right angle.