Answer:
Every value of b>4.47 and b<-4.47 will cause the quadratic equation
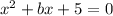 to have two real number solution.
to have two real number solution.
Explanation:
We have the quadratic function
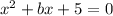 , and we have to find the value of b.
, and we have to find the value of b.
A quadratic function is
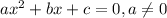 , a quadratic function usually has two real solutions. You can find that solutions using Bhaskara's Formula:
, a quadratic function usually has two real solutions. You can find that solutions using Bhaskara's Formula:
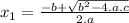
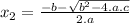
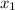 and
and
 are real solutions of the quadratic equation if and only if:
are real solutions of the quadratic equation if and only if:
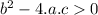
If
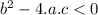 the quadratic equation doesn't have real solutions.
the quadratic equation doesn't have real solutions.
If
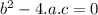 the quadratic equation has only one solution.
the quadratic equation has only one solution.
Then in this case to have two real number solutions:
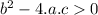
We have
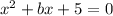 , where a=1, b, c=5
, where a=1, b, c=5
Then,
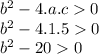
Adding 20 in both sides of the equation:
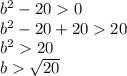
Which is the same as:
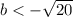
Then,
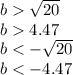
Then every value of b>4.47 and b<-4.47 will cause the quadratic equation
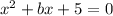 to have two real number solution.
to have two real number solution.
For example b=-5 or b=5.
If you replace with b=-5 in
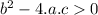
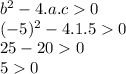
Then the quadratic function has two real number solutions.